Caroline DelbertThu, December 24, 2020, 6:00 AM PST
- A mix of complex and trigonometric values has pinpointed a long-approximated math problem.
- The goat problem represents key ways pure math doesn’t exactly match the “real world.”
- The secret is complex analysis, giving a new frame of reference for the problem.
How do you mix the irrational world of pi with the real world of . . . goats? This is the spirit of a long-unsolved math problem that sounds deceptively simple.
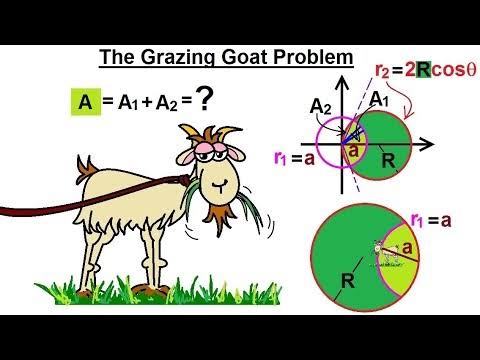
The problem: For a goat to be able to eat grass in a circle with an area of exactly one half acre, how much rope does it need?
The answer has been approximate for centuries, providing food for thought for mathematicians who enjoyed pondering an idle headscratcher. Now, there’s finally a concrete equation.
The goat problem is a living example of what it means to round off your answer. Steve Nadis at Quanta explains the distinction:
“To illustrate the difference, consider the equation x2 − 2 = 0. One could derive an approximate numerical answer, x = 1.4142, but that’s not as accurate or satisfying as the exact solution, x = √2.”

Or consider Zeno’s paradox, the famous thought experiment in which a frog halves its distance across a pond—and literally never gets to the other side. How does Zeno’s chicken cross the road? (It doesn’t.)
With a few moments of thought, the goat problem quickly turns into an exercise in many intersecting approximations. This is why every answer offered since the 1700s has been an approximation as well.
In the 1980s, mathematicians made big progress by blowing out a very hypothetical two dimensions—easy in pure math, impractical in reality—into a 3D space with different mathematics. If this sounds counterintuitive, think about how much of calculus is enabled by switching from x to x2.
And now, finally, there’s an exact solution for the first time. Mathematician Ingo Ullisch took a cue from the previous researchers who made progress on the problem. He introduced complex analysis, which is kind of like algebra with an optional imaginary-number add-on.
By multiplying out a series of values expressed as the telltale a+bi of complex numbers, he was able to reduce the problem to a still-bewildering, but exact expression. Quanta explains the catch:
“Ullisch’s solution is not something simple like the square root of 2. It’s a bit more abstruse—the ratio of two so-called contour integral expressions, with numerous trigonometric terms thrown into the mix—and it can’t tell you, in a practical sense, how long to make the goat’s leash. Approximations are still required to get a number that’s useful to anyone in animal husbandry.”
What’s fun about the goat problem, which mathematicians admit has no relationship to other questions or even mathematical fields, is that it acts as a kind of mathematical Rosetta stone. Whatever your field is, there’s probably a way to approach the problem and model it using your own modeling and analysis.
Something cool about any exact equation is that, technically speaking, it can be set equal to any other exact equation and studied for commonalities.
POPULAR MECHANICS